- Home - Blue Palmetto Home Inspection of Summerville and Charleston
- Learn About Us and This Charleston Home Inspector
- What's Inspected
- Charleston Lowcountry Inspection Areas (geographic)
- Testimonials
- >>>Blogs (educational)
- Sample Reports & Documents
- Why Get a Home Inspection Report
- Charleston's Top Ten Reasons
- Home Inspector Photo Galleries
- Pricing
- Scheduling and Customer Information
- Home Inspection Authorization Contract
- General Scope of Work (electronic)
- Home Inspection Customer Satisfaction Survey
- Privacy Policy
- Full site
- The Roof Framers Field Manual
How to convert the pitch of a roof to degrees
Submitted by Ray Thornburg on Mon, 04/09/2012 - 13:43
How to Figure the Angle of a Roof in Degrees from the Pitch
Tangent is the trigonometric function we use to figure this out. Tangent is the number we get when we divide the opposite side by the adjacent side. Since we are carpenters we’ll call this rise divided by the run or rise/run. For example on a 7/12 roof we divide 7 by 12 to get the number .583333. This number is called the tangent. Next we’ll enter inv tan .5833 and exe on our calculator. The result is 30.255012 or 30 ¼ ˚. Bear in mind that this number is the decimal equivalent so we will have to change it to degrees, minutes and seconds.
Circle = 360 degrees, written as 360˚
Degree = 60 minutes, written as 60’
Minute = 60 seconds, written as 60”
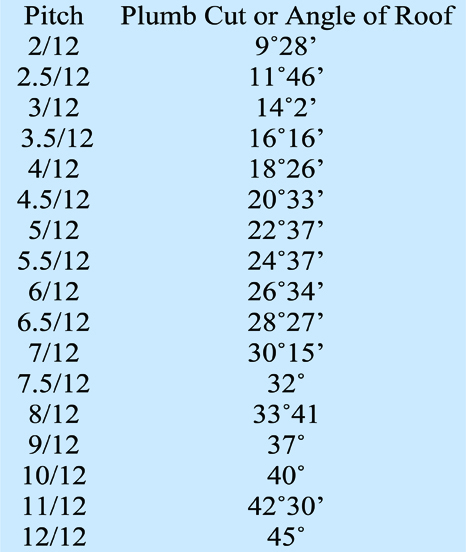
So to change 30.255012 to something we can understand we subtract the integer portion and multiple the decimal portion by 60. For ex. (30.255012 – 30) x 60 = 15.3007 minutes. Now subtract 15 and multiply .3007 x 60 to get 18 seconds. Final answer 7/12 = 30˚15’18”.
It is important to note that most programmable calculators will have a button on it (˚ ‘ “) that will convert decimal degrees to degrees, minutes, seconds automatically.
Common Rafter Terminology
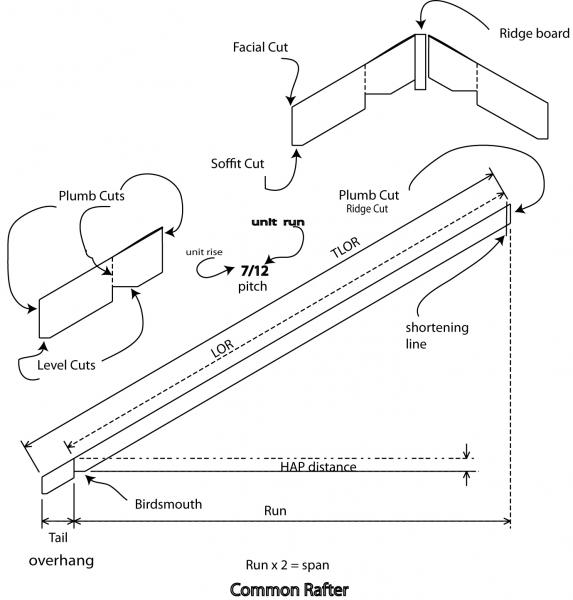
Find a glossary of common rafter terms at our Common Rafter Terminology Page.
For some reason I made two pages with similar information..... so there is more info on this subject at This Page.
At left is a handy conversion chart in case you don't have time for all that math. Click image to enlarge into a new window. The chart shows plumb cuts so remember to get the level cut the formula is 90 - plumb cut = level cut.
Why waste time searching the internet for framing answers piecemeal. My book The Roof Framers Field Manual has it all.

 Click the links to go to my buy now page!
Click the links to go to my buy now page!
If you found this information useful..... Leave a comment...... We"d love to hear from you!